Introduction to P DELTA EFFECTS.

1- Each element has two types of stiffness matrices:
A-Mechanical Stiffness Matrix (Km):
This matrix is based on the physical properties of the element and it is not related to the forces, therefore this matrix can be determined from the first-order analysis step.
B-Geometric/Stress Stiffness Matrix (Kg):
This matrix is a direct function of element’s end joints forces and deformations, thus it couldn’t be determined unless the first-order analysis step is performed, and then extracted forces and deformations are used to formulate the geometric matrix.
The Total Stiffness of the element (Kt) is the total of Mechanical (Km) and Geometric stiffness matrices (Kg).
If the forces extracted from first-order analysis step are compressions, then (Kg) will be negative stiffness, and thus (Kt) will be less than the initial (Km) i.e. (Kt = Km – Kg), meaning that the total stiffness of the element is reduced. And in case that the magnitude of compressive force is so big in a way making Kg > Km, so (Kt) will be negative, and this meaning physically that the structure is unstable.
2- P-Delta effect: is a type of geometric nonlinearity, involves the equilibrium compatibility relationships of a structural system loaded about its deflected configuration.
Of particular concern is the application of gravity load on laterally displaced multi-story building structures. This condition magnifies story drift and certain mechanical behaviors while reducing deformation capacity.
The two sources of P-Delta effect are described as follows:
A- P-Δ effect, or P-"big-delta", is associated with displacements of the member ends. A large P-delta effect is important for overall structure behavior under significant axial load. and To consider P-Δ effect directly, gravity load should be present during nonlinear analysis. The ETABS does a good job of capturing the effect due to the Δ deformation, but it does not typically capture the effect of the δ deformation.
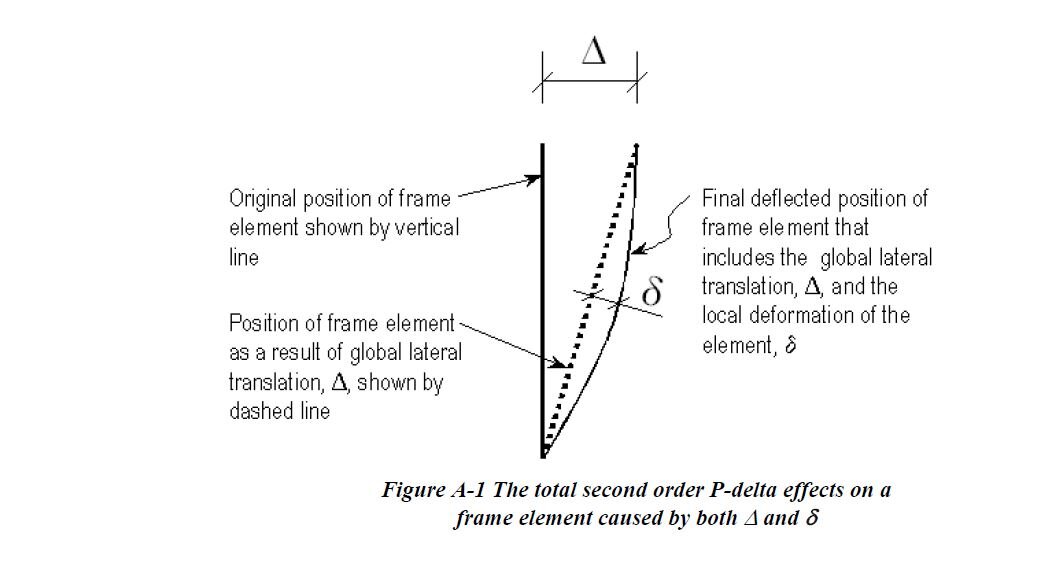
B- P-δ effect, or P-"small-delta", is associated with local deformation relative to the element chord between end nodes. Small P-delta effect is important for local buckling and for design algorithms that expect member buckling to be accounted for by analysis.
In ETAB software, the columns may be subdivided using nodes along their length to evaluate slenderness effects between the ends which is not recommended for concrete elements.
Alternatively, ACI section R 6.7.1.2 allowed that If the column is not subdivided along its length, slenderness effects may be evaluated using the nonsway moment magnifier method specified in 6.6.4.5 (Moment magnification method: Nonsway frames) with member-end moments From the second-order elastic analysis which the same process in ETAB software.
Bear in mind the following issues:
1- If Pu exceeds 0.75Pc the column would be unstable. Hence, if Pu exceeds 0.75Pc , the column cross-section must be enlarged.
2- IF δ exceeds 1.4, ACI code Section 6.2.6 requires that the column cross-section should be enlarged.
3-Sway or not sway
For the purposes of design, a story or a frame can be considered “nonsway” if the moments due to lateral deflections are small compared with the first-order moments due to lateral loads. ACI Code Section 6.6.4.3(a) allows designers to assume that a frame is nonsway if the increase in column-end moments due to second-order effects does not exceed 5 percent of the first-order moments. This can be achieved when the horizontal deflection of one end of a column relative to the other end is prevented, or at least restrained, by walls or other bracing elements.
4- P delta analysis perimeter in ETABS
https://wiki.csiamerica.com/display/etabs/P-Delta+analysis+parameters
Reference :
1. Building code requirements for structural concrete (ACI 318-14) and commentary.
2. Geometric stiffness and p-delta effects by ED Wilson.
3- ETAB software manual.
4- Reinforced concrete Mechanics and Design book.
Thanks to
https://core-en.com/blogs
Er. SP. ASWINPALANIAPPAN., M.E., (Strut/.,)., (Ph.D.,)
Structural Engineer
No comments:
Post a Comment