Analysis of trusses by joint and section method

Numerical problem on the truss Analysis by the method of joints

Analyse the given truss given below by using the method of joints

Step 1: Converting the supports into reactions as shown in the figure below

Step 2: Check for Determinacy

                           w.k.t.

                          m+r-2j=0

                           

                           

                           9+3-2(6) =0

                         

                           Therefore, the structure is determined.

                           

                            Where, 

                            m = The number of members in the structure

           

                            r =   Support Reactions

             

                            j =   Number of joints

Step 3: Calculation of support reactions

               

               Number of unknown reactions = 3 (.i.e. H_A, V_A & V_D)

               Applying the equilibrium conditions

               ΣH = 0

                H_A + 80 = 0 ……….. (1)

                Therefore, H_A = -80 KN

                ΣV = 0

                V_A + V_D = 50KN.............. (2)

               Taking moment about support D

               V_A (3) – 50 (1) + 80 (0.75) = 0............. (3)

               Solving the above equation

               V_A = - 3.33KN

               Substitute V_A in Eq (2)

               Therefore, V_D = 53.33 KN

 Step 4: Free body diagram representing the nature of forces

Step 5: Solving joint A

Applying horizontal equilibrium condition

ΣH = 0

-80 + F_AE + F_AB Cosϴ = 0

(ϴ = Tan^-1(0.75/1))

(ϴ = 36.86^o)

F_AE + F_AB Cos36.86 = 80……… (1)

ΣV = 0

-3.33 + F_AB Sinϴ = 0

F_AB Sin36.86 = 3.33

Therefore, F_AB = 5.55 KN (Tensile)…… (2)

Substitute (2) in (1)

F_AE = 75.56KN(Tensile)

Step 6: Solving joint B

Applying horizontal equilibrium condition

ΣH = 0

F_BC - F_AB Sinα = 0

F_BC – 5.55Sin 53.13 = 0

F_BC = 4.44 KN

ΣV = 0

-F_BE – F_AB Cosα = 0

-F_BE – 5.55Cos 53.13 = 0

 F_BE = -3.33KN

F_BE = 3.33KN (Compression)

Step 7: Solving for joint E

ΣH = 0

- F_AE + F_EF + F_EC Cosϴ = 0…… (1)

ΣV = 0

-F_BE + F_EC Sinϴ = 0

-3.33 + F_EC Sin 36.86 = 0

F_EC = 5.55KN (Tensile) ………. (2)

Substitute (2) in (1)

- F_AE + F_EF + F_EC Cosϴ = 0

-75.56+ F_EF +5.55Cos 36.86 = 0

Therefore, F_EF = 71.12KN(Tensile)

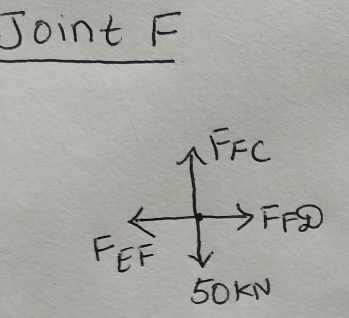
Step 8: Solving for joint F

          ΣH = 0

          

          F_EF = F_FD

        

          F_FD = 71.12KN (Tensile)

          

          ΣV = 0

          

          F_FC = 50KN (Tensile)

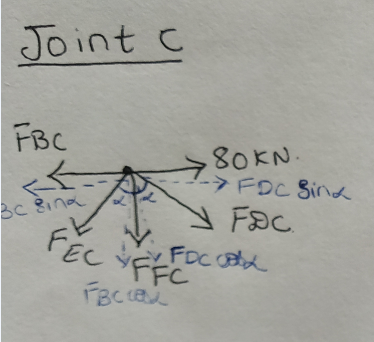
Step 9: Solving for joint C

          ΣH = 0

         -F_BCSinα +80 + F_DC Sinα = 0

         -4.44Sin53.14 + 80 + F_DC Sin53.14 = 0

          F_DC = - 95.55KN

          F_DC = 95.55KN (Compression)

                   Numerical problem on the truss Analysis by the method of Sections

                      Determine the forces in the member's BD, CD & CE

Step 1: Sectioning of a truss

Step 2: Check for Determinacy

          w.k.t.

    m+r-2j=0

. i.e., 7+3-2(5) =0

                                

Therefore, the structure is determined.

Where, 

m = The number of members in the structure

           

 r =   Support Reactions

             

j =   Number of joints

Step 3: Determination of support reactions

Applying the equilibrium conditions

ΣH = 0

Therefore, H_E = 0……… (1)

ΣV = 0

V_A + V_E = 8000 N…….. (2)

Taking moments about E

V_A (2) + 4000 (1) – 1000 (1.5) – 3000(0.5) = 0

Therefore, V_A = 3500N

Substitute (2) in (1)

V_E = 4500N

Step 4: Determination of unknown forces

Taking moment about C

-V_E (1) + 3000(0.5) – F_DB (0.866) = 0

Solving the above equation,

F_DB = -3464 N

F_DB = 3464 N (Compressive)

Applying vertical equilibrium condition

ΣV = 0

-3000 - F_DCSin60 + V_E = 0

Therefore, F_DC = 1732.05N (Tensile)

Taking Moments about D

-V_E (0.5) +F_CE (0.866) = 0

F_CE = 2598N

Er. SP.ASWINPALANIAPPAN., M.E.,(Strut/.,)

Structural Engineer

http://civilbaselife.blogspot.com